what is a vector quantity?

1. Vector Quantity Meaning and Definition
   What is a Vector Quantity?

What is A Vector Quantity?

• A quantity defined by a magnitude and direction with appropriate units is a vector quantity or vector. Some physical quantities that are vector quantities are displacement, velocity, and acceleration.
• The simplest vector quantity is displacement or change of position. A vector representing a displacement is reasonably called a displacement vector. (Similarly, we have velocity vectors and acceleration vectors.)

• Wind velocity is a good example of a vector. When wind velocity is reported, both the magnitude and direction are given, for example, 10 miles per hour out of the north. Vectors require a specified or assumed direction. A vector quantity is incomplete without a direction.
• The importance of including the direction for a vector can be illustrated by considering a person standing at the end of a narrow dock.
  If the person were told to move three steps forward versus three steps directly backward, it would probably make the difference between falling in the water and staying dry. Three steps is a scalar, whereas three steps backward is a vector.

2. Some Laws for Vector Quantity

Some of the laws are related to vector quantity.

1. MULTIPLICATION BY SCALAR
2. COMMUTATIVITY OF ADDITION
3. COMMUTATIVITY OF VECTOR-SCALAR MULTIPLICATION
4. COMMUTATIVITY OF DOT PRODUCT
5. NEGATIVE COMMUTATIVITY OF CROSS PRODUCT
6. ASSOCIATIVITY OF ADDITION
7. ASSOCIATIVITY OF VECTOR-SCALAR MULTIPLICATION
8. DISTRIBUTIVITY OF SCALAR MULTIPLICATION OVER SCALAR ADDITION
9. DISTRIBUTIVITY OF SCALAR MULTIPLICATION OVER VECTOR ADDITION
10. DISTRIBUTIVITY OF DOT PRODUCT OVER VECTOR ADDITION
11. DISTRIBUTIVITY OF CROSS PRODUCT OVER VECTOR ADDITION
12. DOT PRODUCT OF CROSS PRODUCTS

1. MULTIPLICATION BY SCALAR

• When any vector is multiplied by a real number, also known as a scalar, the vector magnitude (length) is multiplied by that scalar. The direction remains unchanged if the scalar is positive but is reversed if the scalar is negative.

2. COMMUTATIVITY OF ADDITION

• When you add two vectors, it does not matter in which order the sum is per- formed. If a and b are vectors, then
  a+b=b+a

3. COMMUTATIVITY OF VECTOR-SCALAR MULTIPLICATION

• When a vector is multiplied by a scalar, it does not matter in which order the product is performed. If a is a vector and k is a real number, then
  ka = ak

4. COMMUTATIVITY OF DOT PRODUCT

• When the dot product of two vectors is found, it does not matter in which order the vectors are placed. If a and b are vectors, then
  a.b = b.a

5. NEGATIVE COMMUTATIVITY OF CROSS PRODUCT

• The cross-product of two vectors reverses direction when the order in which the vectors are “multiplied” is reversed. That is,
  b ✖️ a = – (a ✖️ b)

6. ASSOCIATIVITY OF ADDITION

• When you add three vectors, it makes no difference how the sum is grouped. If a, b, and c are vectors, then
  (a + b) + c = a + (b + c)

7. ASSOCIATIVITY OF VECTOR-SCALAR MULTIPLICATION

• Let a be a vector, and let k1 and k2 be real-number scalars. Then the following equation holds:
  k₁ (k₂ a) = (k₁ k₂) a

8. DISTRIBUTIVITY OF SCALAR MULTIPLICATION OVER SCALAR ADDITION

• Let a be a vector, and let k1 and k2 be real-number scalars. Then the following equations hold:
  (k₁ + k₂) a = k₁ a + k₂ a
  a (k₁ + k₂) = a k₁ + a k₂ = k₁ a + k₂ a

9. DISTRIBUTIVITY OF SCALAR MULTIPLICATION OVER VECTOR ADDITION

• Let a and b be vectors, and let k be a real-number scalar. Then the follow- ing equations hold:
  k (a + b) = k a + k b
  (a + b) k = a k + b k = k a + k b

10. DISTRIBUTIVITY OF DOT PRODUCT OVER VECTOR ADDITION

• Let a, b, and c be vectors. Then the following equations hold:
  a . (b + c) = a . b + a . c
  (b + c) . a = b . a + c . a = a . b + a . c

11. DISTRIBUTIVITY OF CROSS PRODUCT OVER VECTOR ADDITION

• Let a, b, and c be vectors. Then the following equations hold:
  a ✖️ (b + c) = a ✖️ b + a ✖️ c
  
  (b + c) ✖️ a = b ✖️ a + c ✖️ a
                     = -(a ✖️ b) – (a ✖️ c)
                     = -(a ✖️ b + a ✖️ c)

12. DOT PRODUCT OF CROSS PRODUCTS

• Let a, b, c, and d be vectors. Then the following equation holds:
  (a ✖️ b) . (c ✖️ d) = (a . c) (b . d) – (a . d) (b . c)

These are only a few examples of the rules vectors universally obey.

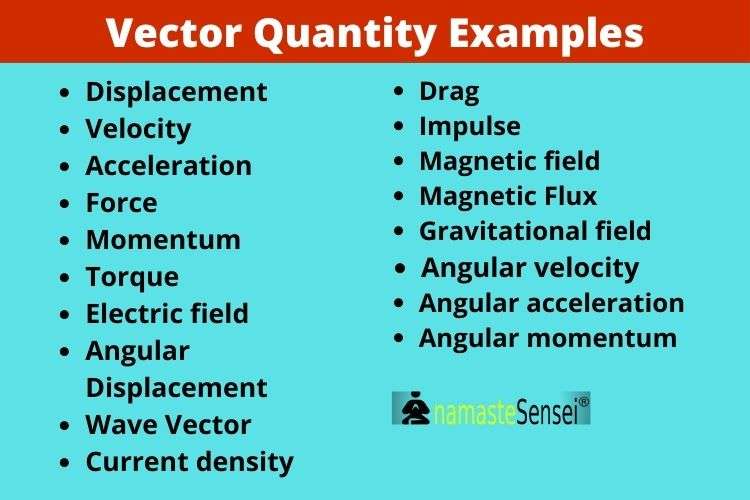
3. Some Examples of Vector Quantity in physics

Examples

Following are some examples

1. Displacement

• The change in the position of a moving body in a particular direction is called its displacement or the shortest distance between the final and the initial positions of a body are called displacement. It is generally denoted by D or X. Displacement is a vector quantity.
• For example – If an object moves from A position to B, then the object’s position changes.

2. Velocity

• The distance traveled by a body in a particular direction in unit time is called its velocity. velocity is a vector quantity mark.
• SI unit for velocity is meter per second (m/s).
• For example, the speed of a car traveling north on a highway, or the speed a rocket travels after launching.

3. Acceleration

• The time rate of change of velocity of a body is called acceleration.
• Acceleration = Change in Velocity / Time taken
• SI unit for accleretaion is meter per second square (m/s²).
• For example, the speed of a car traveling north on a highway, or the speed a rocket travels after launching.

4. Force

• Force is a push or pull that changes or tends to change the state of rest or of uniform motion, the direction of motion, or the shape and size of a body.
• Force is a vector quantity.
• The SI unit of force is Newton. It is denoted by N.
  For example, a force of 5 Newton may be described as 5 N.

5. Momentum

• The product of the mass of a body and its velocity is called the momentum of the body
• It is generally denoted by ‘p’.
• SI unit of momentum will be kgm/s^-1.

6. Torque

• Torque measures the force that can cause an object to rotate about an axis.
• Torque is commonly denoted with a capital “T”. It is spelled as (tau).
• SI unit of torque is newton meter (Nm)

7. Electric field

• The electric field is the ratio of force per unit charge. Since force is a vector quantity, the electric field is also a vector quantity. It is denoted by ‘E’.
• The SI unit of the electric field is volts per meter (V/m).
• Electric Field (E) = Force(F) / charge(q), where E is the strength of the electric field, F is the electric force, and q is the test charge that is being used to “feel” the electric field.

Read more Examples in this linked article

Recommended Read – Examples Of Vector Quantities

Vector quantity examples

Congratulations, you have read the complete article about vector quantity. If you have any doubts or queries, feel free to comment below. We will respond as soon as possible.

Or Email Us At [email protected]

More Articles:

30 Examples Of Vector Quantity

Difference Between Scalar And Vector Quantity

Any topic you want us to cover? Let us know.